How to Calculate Diameter From Circumference: A Simple Guide
Knowing how to calculate the diameter from the circumference is a fundamental skill in mathematics and various practical applications. Whether you're working on a geometry problem, designing a circular object, or simply curious about the relationship between these two measurements, this guide will provide you with a clear understanding of the process and the formula involved.
Understanding the Relationship Between Diameter and Circumference
Before diving into the calculation, let's clarify the definitions:
-
Diameter: The diameter of a circle is the straight line passing through the center of the circle and connecting two points on the opposite edge. It's essentially the longest chord of the circle.
-
Circumference: The circumference of a circle is the distance around the circle. Think of it as the perimeter of a circular shape.
The relationship between these two is constant and defined by the mathematical constant π (pi), approximately equal to 3.14159. The formula linking them is:
Circumference = π * Diameter
Calculating Diameter from Circumference: The Formula
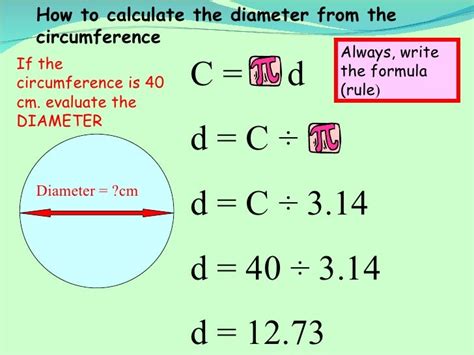
To find the diameter when you only know the circumference, we need to rearrange the above formula. We isolate the diameter (D) by dividing both sides of the equation by π:
Diameter (D) = Circumference / π
Step-by-Step Calculation
Let's illustrate this with an example. Suppose you have a circle with a circumference of 25 centimeters. Here's how to calculate its diameter:
-
Identify the circumference: Circumference = 25 cm
-
Apply the formula: Diameter (D) = Circumference / π
-
Substitute the value: D = 25 cm / 3.14159
-
Calculate the result: D ≈ 7.96 cm
Therefore, the diameter of the circle is approximately 7.96 centimeters.
Practical Applications
Understanding this calculation is crucial in various fields:
-
Engineering and Design: Calculating diameters is essential when designing circular components for machinery, buildings, or other structures.
-
Construction: Determining the diameter of pipes, columns, or other circular elements is critical for accurate measurements and efficient construction.
-
Everyday Life: From measuring the diameter of a pizza to understanding the size of a circular garden bed, this calculation has practical applications in everyday scenarios.
Tips for Accurate Calculations
-
Use a precise value of π: For greater accuracy, use the full value of π provided by your calculator or software, rather than a rounded approximation like 3.14.
-
Double-check your units: Ensure your circumference measurement is in consistent units (e.g., centimeters, inches, meters) for an accurate diameter calculation.
-
Consider significant figures: When dealing with measurements, pay attention to significant figures to avoid unnecessary precision in your final answer.
By mastering this simple formula, you gain a valuable tool for solving problems related to circles and their dimensions. Remember, the key is understanding the relationship between the circumference and the diameter, linked inseparably through the fascinating constant, π.