Introduction: Why Bother with Page Numbers?
In the digital age, it’s easy to overlook the importance of proper document formatting. But when it comes to creating professional, organized documents, page numbers are non-negotiable. Imagine a lengthy report without them – a chaotic mess of information that’s impossible to navigate. Whether you’re crafting a thesis, a business proposal, or even a simple essay, adding page numbers is a crucial step in ensuring clarity and readability. This guide will walk you through the process of adding page numbers to your Word documents, specifically focusing on the footer, offering tips and tricks for customization along the way.
Why the footer, you ask? Well, the footer is a discreet and consistent location, ensuring that your page numbers are visible without being intrusive. It’s a standard practice in document design, making your work look polished and professional. So, let’s dive in and explore the various ways to add and customize page numbers in Word’s footer section.
Understanding the Basics: Accessing the Header & Footer in Word
Before we delve into the specifics of adding page numbers, it’s essential to understand how to access the header and footer areas in Microsoft Word. These sections reside at the top and bottom margins of each page, respectively, and are designed for content that you want to repeat throughout the document, such as page numbers, titles, dates, or company logos.
Methods to Access Header & Footer:
- Double-Clicking: The simplest method is to double-click near the top or bottom of the page. This will automatically open the header or footer area for editing. Word will visually indicate that you are now in the header/footer mode by dimming the main document content.
- Insert Tab: Alternatively, you can navigate to the ‘Insert’ tab on the Word ribbon. In the ‘Header & Footer’ group, you’ll find dedicated buttons for ‘Header’ and ‘Footer’. Clicking these buttons will reveal a dropdown menu with various pre-designed header and footer styles, as well as an option to ‘Edit Header’ or ‘Edit Footer’.
- Layout Tab (Page Setup): In some versions of Word, you may find options related to headers and footers within the ‘Layout’ tab (or ‘Page Layout’ in older versions). Look for the ‘Page Setup’ group, and you might find a launcher button (a small arrow in the corner) that opens the Page Setup dialog box. Within this dialog, there might be a ‘Layout’ tab where you can adjust header and footer margins. While this doesn’t directly open the header/footer for editing, it allows you to control their size and position.
Once you’ve accessed the header or footer, a new ‘Header & Footer Tools Design’ tab will appear on the ribbon. This tab contains all the necessary tools for inserting page numbers, dates, times, logos, and other elements. Familiarizing yourself with these tools is crucial for effectively managing your document’s header and footer sections.
Adding Basic Page Numbers to the Footer: A Step-by-Step Guide
Now, let’s get to the core of the matter: adding page numbers to the footer. This is a straightforward process, and Word offers several convenient options to achieve this.
Step-by-Step Instructions:
- Access the Footer: Use one of the methods described above (double-clicking or the ‘Insert’ tab) to open the footer area of your document.
- Navigate to the ‘Header & Footer Tools Design’ Tab: Ensure that this tab is active. It should appear automatically when you’re in the header or footer area.
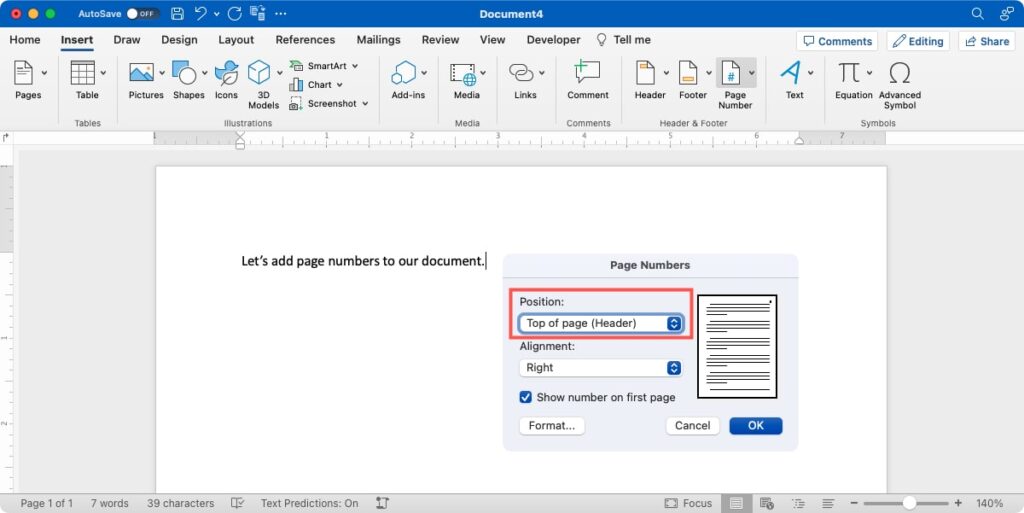
- Click ‘Page Number’: In the ‘Header & Footer’ group on the ribbon, click the ‘Page Number’ button. This will open a dropdown menu with various options for page number placement.
- Choose ‘Bottom of Page’: Hover over the ‘Bottom of Page’ option. This will reveal a submenu with different styles for placing the page number in the footer.
- Select a Style: Choose a style that suits your preferences. You’ll find options like ‘Plain Number 1’ (left-aligned), ‘Plain Number 2’ (centered), ‘Plain Number 3’ (right-aligned), and more elaborate styles with lines or other design elements. Click on your desired style to insert the page number into the footer.
Word will automatically insert the current page number into the footer of the current page and subsequent pages. The page numbers will update automatically as you add or remove pages from your document. It’s that simple!
Customizing Your Page Numbers: Beyond the Basics
While adding basic page numbers is easy, Word offers a wealth of customization options to tailor the appearance and behavior of your page numbers to your specific needs. Let’s explore some of these options.
Changing the Number Format:
By default, Word uses Arabic numerals (1, 2, 3, …) for page numbers. However, you can easily change this to Roman numerals (I, II, III, …), letters (a, b, c, …), or other formats.
- Access the ‘Page Number Format’ Dialog: In the ‘Header & Footer Tools Design’ tab, click the ‘Page Number’ button again, and then select ‘Format Page Numbers…’. This will open the ‘Page Number Format’ dialog box.
- Choose a Number Format: In the ‘Number format’ dropdown menu, select your desired format (e.g., ‘i, ii, iii, …’ for lowercase Roman numerals).
- Adjust Starting Number: If you want your page numbering to start at a number other than 1, enter the desired starting number in the ‘Start at’ field. This is useful for documents with introductory pages that shouldn’t be numbered.
- Click ‘OK’: Click ‘OK’ to apply the changes.
Adding Chapter Numbers:
For longer documents, you might want to include chapter numbers along with page numbers. This helps readers understand the context of each page.
- Enable Chapter Numbering: In the ‘Page Number Format’ dialog box (accessed as described above), check the ‘Include chapter number’ box.
- Choose a Chapter Style: In the ‘Chapter starts with style’ dropdown menu, select the heading style that you use to define your chapters (e.g., ‘Heading 1’).
- Choose a Separator: In the ‘Separator’ dropdown menu, select a character to separate the chapter number from the page number (e.g., a hyphen or an em dash).
- Click ‘OK’: Click ‘OK’ to apply the changes.
Adding Text Before or After the Page Number:
You can also add custom text before or after the page number. This is useful for adding prefixes like ‘Page’ or suffixes like ‘- [Document Title]’.
- Insert the Page Number Field: First, insert the basic page number field into the footer as described earlier.
- Add Text: Then, simply type the desired text before or after the page number field. For example, you could type ‘Page ‘ before the page number or ‘ – [Your Name]’ after it.
Removing Page Numbers from the First Page:
In some cases, you might want to omit the page number from the first page of your document, such as a title page or a cover page.
- Enable ‘Different First Page’: In the ‘Header & Footer Tools Design’ tab, check the ‘Different First Page’ box.
- Delete the Page Number on the First Page: This will create a separate header and footer for the first page. Simply delete the page number field from the footer of the first page. The page numbers on subsequent pages will remain intact.
Linking and Unlinking Headers and Footers:
Word allows you to link or unlink headers and footers between sections of your document. This is useful for creating different headers and footers for different parts of your document, such as chapters or appendices.
- Insert Section Breaks: First, insert section breaks into your document where you want the header and footer to change. Go to the ‘Layout’ tab, click ‘Breaks’, and choose the appropriate type of section break (e.g., ‘Next Page’ or ‘Continuous’).
- Unlink the Header/Footer: Go to the header or footer of the section where you want to make changes. In the ‘Header & Footer Tools Design’ tab, the ‘Link to Previous’ button will be highlighted if the header/footer is linked to the previous section. Click the ‘Link to Previous’ button to unlink it.
- Customize the Header/Footer: Now you can customize the header or footer of the current section without affecting the headers and footers of other sections.
Troubleshooting Common Page Numbering Issues
Even with a clear understanding of the process, you might encounter some common issues when working with page numbers in Word. Here’s a troubleshooting guide to help you resolve them.
Page Numbers Not Updating:
Sometimes, page numbers might not update automatically after you add or remove pages. This can be frustrating, but it’s usually an easy fix.
- Manual Update: Try manually updating the page numbers by selecting all the text in your document (Ctrl+A) and then pressing F9. This will force Word to recalculate and update all fields, including page numbers.
- Check Field Codes: Ensure that the page number is inserted as a field code. To check, right-click on the page number and select ‘Toggle Field Codes’. You should see something like ‘{ PAGE }’. If you see plain text instead, you’ll need to re-insert the page number using the ‘Page Number’ button in the ‘Header & Footer Tools Design’ tab.
Incorrect Starting Page Number:
If your page numbering starts at the wrong number, you can adjust the starting number as described earlier in the customization section.
- Access ‘Format Page Numbers’: Go to the ‘Header & Footer Tools Design’ tab, click ‘Page Number’, and select ‘Format Page Numbers…’.
- Adjust ‘Start at’: In the ‘Page Number Format’ dialog box, enter the desired starting number in the ‘Start at’ field.
Page Numbers Appearing on Unwanted Pages:
If you want to remove page numbers from specific pages, such as the first page or a blank page, use the ‘Different First Page’ option or insert section breaks to create separate headers and footers for those pages.
- ‘Different First Page’: In the ‘Header & Footer Tools Design’ tab, check the ‘Different First Page’ box to create a separate header and footer for the first page.
- Section Breaks: Insert section breaks before and after the page you want to exclude from page numbering. Then, unlink the header/footer of that section from the previous section and delete the page number.
Page Numbers Overlapping with Other Content:
If your page numbers are overlapping with other content in the footer, you might need to adjust the footer margins or the position of the page number field.
- Adjust Footer Margins: Go to the ‘Layout’ tab (or ‘Page Layout’ in older versions), click the ‘Margins’ button, and select ‘Custom Margins…’. In the ‘Page Setup’ dialog box, adjust the ‘Bottom’ margin to increase the space for the footer.
- Reposition Page Number Field: Simply click and drag the page number field within the footer to reposition it. You can also use the alignment tools in the ‘Home’ tab to align the page number to the left, center, or right.
Advanced Techniques: Working with Multiple Sections and Complex Documents
For complex documents with multiple sections, such as books or reports with appendices, you’ll need to master advanced techniques for managing page numbers. This involves using section breaks and linking/unlinking headers and footers strategically.
Using Section Breaks:
Section breaks allow you to divide your document into distinct sections, each with its own formatting settings, including headers and footers. There are several types of section breaks:
- Next Page: Starts the new section on the next page.
- Continuous: Starts the new section on the same page.
- Even Page: Starts the new section on the next even-numbered page.
- Odd Page: Starts the new section on the next odd-numbered page.
To insert a section break, go to the ‘Layout’ tab, click ‘Breaks’, and choose the appropriate type of section break.
Linking and Unlinking Headers and Footers (Revisited):
As mentioned earlier, you can link or unlink headers and footers between sections. When a header/footer is linked, it inherits the content and formatting of the previous section’s header/footer. When it’s unlinked, you can customize it independently.
To unlink a header/footer, go to the header or footer of the section you want to modify. In the ‘Header & Footer Tools Design’ tab, click the ‘Link to Previous’ button to toggle the linking status.
Example: Numbering Chapters and Appendices Separately:
Let’s say you want to number the chapters of your document with Arabic numerals (1, 2, 3, …) and the appendices with Roman numerals (A, B, C, …).
- Insert Section Breaks: Insert a ‘Next Page’ section break before the first appendix.
- Unlink the Footer of the Appendix Section: Go to the footer of the first page of the appendix section. In the ‘Header & Footer Tools Design’ tab, click the ‘Link to Previous’ button to unlink it.
- Change the Number Format for the Appendix Section: In the ‘Header & Footer Tools Design’ tab, click ‘Page Number’, select ‘Format Page Numbers…’, and choose ‘A, B, C, …’ from the ‘Number format’ dropdown menu. Also, set the ‘Start at’ value to ‘A’.
- Verify Chapter Numbering: Ensure that the chapter section continues to use Arabic numerals and that the numbering is sequential.
By using section breaks and linking/unlinking headers and footers, you can create complex and professionally formatted documents with customized page numbering schemes.
Best Practices for Page Numbering in Word
To ensure that your page numbering is consistent, accurate, and visually appealing, follow these best practices:
- Choose a Consistent Style: Select a page number style that complements the overall design of your document and use it consistently throughout.
- Use Section Breaks Wisely: Use section breaks to divide your document into logical sections with distinct formatting requirements.
- Double-Check Your Work: Before finalizing your document, carefully review all page numbers to ensure that they are accurate and properly formatted.
- Consider Your Audience: Think about your audience and choose a page numbering scheme that is easy for them to understand and navigate.
- Accessibility: Ensure that your page numbers are visible and legible for all readers, including those with visual impairments. Use a font size and color that provide sufficient contrast with the background.
Conclusion: Mastering Page Numbering for Professional Documents
Adding page numbers to the footer in Microsoft Word is a fundamental skill for creating professional and organized documents. While the basic process is simple, Word offers a wealth of customization options to tailor the appearance and behavior of your page numbers to your specific needs. By understanding the basics of accessing headers and footers, customizing number formats, and troubleshooting common issues, you can ensure that your documents are easy to navigate and visually appealing.
From simple essays to complex reports with multiple sections, mastering page numbering techniques is essential for presenting your work in a polished and professional manner. So, take the time to explore the various options available in Word and practice these techniques until they become second nature. Your readers (and your grade!) will thank you for it.

