How to Calculate the Surface Area of a Cube: A Simple Guide
Calculating the surface area of a cube is a fundamental concept in geometry with applications in various fields, from packaging design to construction. This guide provides a clear, step-by-step approach to mastering this calculation, complete with examples and tips for accuracy.
Understanding the Cube
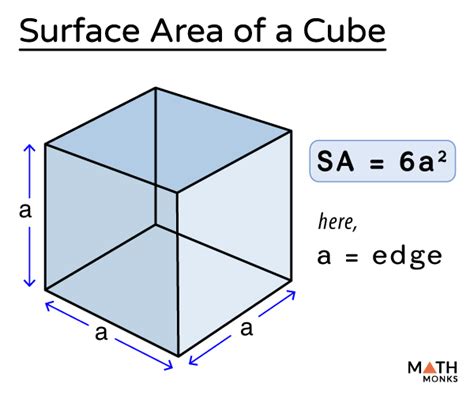
A cube is a three-dimensional solid object bounded by six square faces, facets or sides, with three meeting at each vertex. All of its sides are equal in length. This uniformity simplifies the surface area calculation considerably.
The Formula for Surface Area of a Cube
The surface area of any object is simply the total area of all its surfaces. Since a cube has six identical square faces, the formula is elegantly simple:
Surface Area = 6 * side²
Where:
- Surface Area represents the total surface area of the cube.
- side represents the length of one side (or edge) of the cube. All sides are equal in length.
Step-by-Step Calculation
Let's break down the calculation process with a practical example:
Example: Imagine a cube with each side measuring 5 centimeters (cm).
Step 1: Identify the side length.
In our example, the side length (side) is 5 cm.
Step 2: Square the side length.
Square the side length: 5 cm * 5 cm = 25 cm²
Step 3: Multiply by 6.
Multiply the squared side length by 6 (the number of faces): 25 cm² * 6 = 150 cm²
Step 4: State the result.
The surface area of the cube is 150 square centimeters.
Practical Applications
Understanding how to calculate the surface area of a cube is crucial in many real-world scenarios, including:
- Packaging: Determining the amount of material needed to create a box.
- Construction: Calculating the amount of paint or siding required for a cubic structure.
- Engineering: Analyzing the surface area to volume ratio in various designs.
Tips for Accurate Calculation
- Consistent Units: Always use the same units of measurement throughout your calculation (e.g., centimeters, meters, inches).
- Precise Measurement: Ensure accurate measurement of the cube's side length. Inaccurate measurements will lead to inaccurate surface area calculations.
- Double-Check Your Work: Review your calculations to avoid simple mathematical errors.
Beyond the Basics: Exploring Related Concepts
While this guide focuses on the basic calculation, understanding cube surface area opens doors to more complex geometrical problems. Further exploration might include:
- Volume of a Cube: Learning how to calculate the volume (space inside) of a cube.
- Surface Area of other 3D Shapes: Extending your knowledge to calculate the surface areas of prisms, pyramids, and other polyhedrons.
Mastering the calculation of a cube's surface area is a foundational step in understanding geometry and its practical applications. By following these steps and tips, you can confidently tackle this calculation and build a strong foundation for more advanced geometrical concepts.