How to Calculate Volume: A Comprehensive Guide
Understanding how to calculate volume is a fundamental skill with applications across various fields, from everyday tasks to complex scientific calculations. This guide will walk you through different methods for calculating volume, catering to various shapes and scenarios. We'll cover the basics and provide practical examples to solidify your understanding.
Understanding Volume
Before diving into the calculations, let's define what volume is. Volume is the amount of three-dimensional space occupied by an object or substance. It's often measured in cubic units, such as cubic centimeters (cm³), cubic meters (m³), cubic feet (ft³), or liters (L).
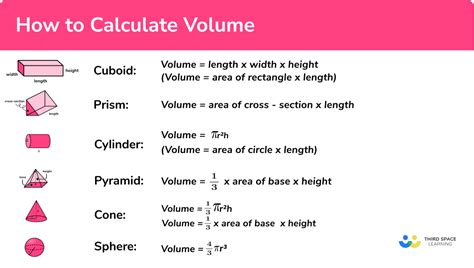
Calculating Volume for Common Shapes
The method for calculating volume depends heavily on the shape of the object. Here are some common shapes and their respective formulas:
1. Cube
A cube is a three-dimensional shape with six square faces of equal size. Calculating its volume is straightforward:
Formula: Volume = side³ (side cubed)
- Where: "side" represents the length of one side of the cube.
Example: A cube with sides of 5 cm has a volume of 5 cm * 5 cm * 5 cm = 125 cm³.
2. Rectangular Prism (Cuboid)
A rectangular prism, also known as a cuboid, is a three-dimensional shape with six rectangular faces.
Formula: Volume = length × width × height
Example: A rectangular prism with a length of 10 cm, a width of 5 cm, and a height of 3 cm has a volume of 10 cm × 5 cm × 3 cm = 150 cm³.
3. Cylinder
A cylinder is a three-dimensional shape with two parallel circular bases and a curved surface connecting them.
Formula: Volume = π × radius² × height
- Where: π (pi) ≈ 3.14159, "radius" is the distance from the center of the circular base to its edge, and "height" is the distance between the two bases.
Example: A cylinder with a radius of 4 cm and a height of 10 cm has a volume of approximately 3.14159 × 4 cm² × 10 cm ≈ 502.65 cm³.
4. Sphere
A sphere is a perfectly round three-dimensional object.
Formula: Volume = (4/3) × π × radius³
Example: A sphere with a radius of 6 cm has a volume of approximately (4/3) × 3.14159 × 6 cm³ ≈ 904.78 cm³.
5. Cone
A cone is a three-dimensional shape with a circular base and a single vertex.
Formula: Volume = (1/3) × π × radius² × height
6. Irregular Shapes
Calculating the volume of irregular shapes requires different techniques. One common method is water displacement. Submerge the object in a container of water and measure the amount of water displaced. The volume of the displaced water equals the volume of the object.
Tips for Accurate Volume Calculations
- Use consistent units: Ensure all measurements are in the same units before performing calculations.
- Double-check your measurements: Inaccurate measurements lead to inaccurate volume calculations.
- Use a calculator: For more complex calculations, utilize a calculator to ensure accuracy.
- Understand the formulas: Familiarize yourself with the formulas for various shapes before attempting calculations.
Conclusion
Mastering volume calculations is a valuable skill applicable in numerous situations. By understanding the formulas and applying the tips mentioned above, you'll be able to accurately determine the volume of various objects, regardless of their shape. Remember to always double-check your work and choose the appropriate formula for the shape you're working with.