How to Find Slope: A Comprehensive Guide
Finding the slope of a line is a fundamental concept in algebra and geometry. Understanding slope allows you to analyze the steepness and direction of a line, which is crucial for various applications in mathematics and beyond. This guide will walk you through different methods of calculating slope, ensuring you master this essential skill.
What is Slope?
Slope, often represented by the letter 'm', describes the steepness and direction of a line. It's the ratio of the vertical change (rise) to the horizontal change (run) between any two distinct points on a line. A positive slope indicates an upward trend from left to right, while a negative slope indicates a downward trend. A slope of zero represents a horizontal line, and an undefined slope indicates a vertical line.
Methods for Finding Slope
There are several ways to determine the slope of a line, depending on the information you have available.
1. Using Two Points
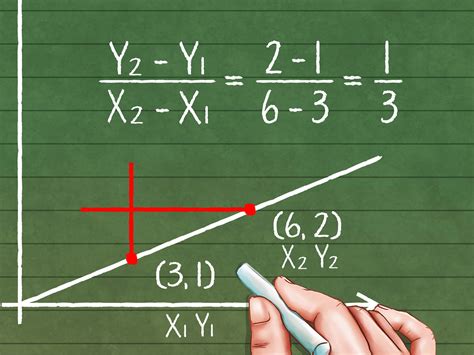
This is the most common method. If you know the coordinates of two points on the line, (x₁, y₁) and (x₂, y₂), you can calculate the slope using the following formula:
m = (y₂ - y₁) / (x₂ - x₁)
Example: Find the slope of the line passing through points (2, 3) and (5, 9).
- Identify your points: (x₁, y₁) = (2, 3) and (x₂, y₂) = (5, 9)
- Apply the formula: m = (9 - 3) / (5 - 2) = 6 / 3 = 2
Therefore, the slope of the line is 2.
Important Note: Ensure you subtract the y-coordinates and x-coordinates in the same order. Inconsistency will lead to an incorrect slope.
2. Using the Equation of a Line
If the equation of the line is in slope-intercept form (y = mx + b), where 'm' is the slope and 'b' is the y-intercept, the slope is simply the coefficient of 'x'.
Example: Find the slope of the line y = 2x + 5.
The slope 'm' is 2.
3. Using a Graph
If you have a graph of the line, you can find the slope by selecting two points on the line and counting the rise (vertical change) and the run (horizontal change) between them.
Example: Visually inspect the graph. If you move 2 units up (rise) and 1 unit to the right (run) between two points, the slope is 2/1 = 2.
Understanding Different Slopes
- Positive Slope: The line rises from left to right.
- Negative Slope: The line falls from left to right.
- Zero Slope: The line is horizontal.
- Undefined Slope: The line is vertical.
Applications of Slope
Understanding slope is essential in various fields:
- Engineering: Calculating gradients for roads and structures.
- Physics: Determining velocity and acceleration.
- Data Analysis: Interpreting trends and relationships in data.
Conclusion
Mastering how to find slope is a crucial skill for anyone studying mathematics or related fields. By understanding the different methods and interpretations, you can confidently analyze and interpret linear relationships in various contexts. Remember to practice regularly to solidify your understanding and build your problem-solving abilities.