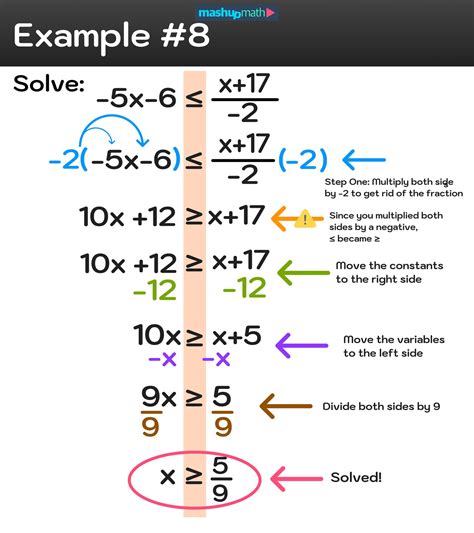
How to Solve Inequalities: A Comprehensive Guide
Inequalities, those mathematical statements showing that two expressions are not equal, can seem daunting at first. But with a structured approach and understanding of the core principles, solving inequalities becomes significantly easier. This guide provides a step-by-step process to tackle various types of inequalities, equipping you with the skills to confidently solve them.
Understanding Inequality Symbols
Before diving into solutions, let's refresh our understanding of the inequality symbols:
- >: Greater than
- <: Less than
- ≥: Greater than or equal to
- ≤: Less than or equal to
- ≠: Not equal to
These symbols dictate the relationship between the two expressions being compared.
Solving Linear Inequalities
Linear inequalities involve variables raised to the power of one. Solving them follows a similar process to solving linear equations, with one crucial difference: when multiplying or dividing by a negative number, you must reverse the inequality sign.
Example: Solve 3x + 5 < 11
- Subtract 5 from both sides: 3x < 6
- Divide both sides by 3: x < 2
The solution is x < 2. This means any value of x less than 2 satisfies the inequality.
Solving Compound Inequalities
Compound inequalities involve two or more inequalities combined using "and" or "or."
Example (And): Solve -2 ≤ 2x + 4 ≤ 6
- Subtract 4 from all parts: -6 ≤ 2x ≤ 2
- Divide all parts by 2: -3 ≤ x ≤ 1
The solution is -3 ≤ x ≤ 1, meaning x can be any value between -3 and 1, inclusive.
Example (Or): Solve x + 2 > 5 or x - 3 < -1
- Solve each inequality separately:
- x + 2 > 5 => x > 3
- x - 3 < -1 => x < 2
- Combine the solutions: x > 3 or x < 2
The solution is x > 3 or x < 2.
Solving Quadratic Inequalities
Quadratic inequalities involve variables raised to the power of two. Solving these requires finding the roots of the corresponding quadratic equation and testing intervals.
Example: Solve x² - 4x - 5 < 0
- Find the roots of x² - 4x - 5 = 0: (x - 5)(x + 1) = 0; x = 5 or x = -1
- Test intervals: Choose a value from each interval (-∞, -1), (-1, 5), and (5, ∞) and substitute it into the inequality.
- Determine the solution: The inequality is true when x is between -1 and 5.
The solution is -1 < x < 5.
Graphing Inequalities
Visualizing inequalities on a number line provides a clear representation of the solution. Use open circles for < and >, and closed circles for ≤ and ≥.
Example: Graphing x < 2
You would draw a number line, place an open circle at 2, and shade the region to the left of 2.
Tips for Success
- Practice regularly: The more you practice, the more comfortable you'll become with solving different types of inequalities.
- Check your work: Always substitute your solution back into the original inequality to ensure it's correct.
- Use online resources: Many websites and videos offer further explanations and practice problems.
- Break down complex problems: If you encounter a particularly difficult inequality, break it down into smaller, more manageable steps.
By following these steps and practicing consistently, you'll master the art of solving inequalities and confidently tackle any mathematical challenges they present. Remember, understanding the underlying principles is key to success.